Divide Mononomials
Algebra Help: Section 2.19
Learn to divide mononomials with algebra.
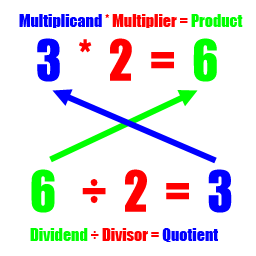
Division is the process of finding how many times one quantity is contained in another. Division's the converse of multiplication. The dividend corresponds to the product. The divisor and quotient correspond to the multiplier and multiplicand.
However in multiplication, the order of multiplicand and multiplier don't matter. In division, the order of dividend and divisor do matter. Factors are commutative in multiplication but not in division.
Dividend ÷ Divisor
= Quotient
EXAMPLE DIVISION
6 ÷ 2 = 3
DIVISION: FRACTION BAR
Dividend 6
___ = 3 Quotient
Divisor 2
Multiplicand * Multiplier
= Product
EXAMPLE MULTIPLICATION
3 * 2 = 6
Multiplicand
corresponds to
Quotient
Multiplier
corresponds to
Divisor
Product
corresponds to
Dividend
Case I
1. Both dividend and divisor are mononomials.
Divide 6ab by 2a.
OPERATION
6ab ÷ 2a = 3b
ANALYSIS -
Since division is the converse
of multiplication, look for
a quantity, which when multiplied by 2a
will produce 6ab.
The quantity, 3b is found by
dividing 6 by 2.
Drop the factor a, because
a ÷ a equals 1.
Just as any number divided by itself equals 1.
2. Divide x5 by x3.
OPERATION:
x5 ÷ x3 = x2
ANALYSIS - When multiplying add exponents. When dividing subtract exponents. Therefore subtract exponents 3 from 5. The difference equals 2. The exponent of the quotient equals 2.
NOTE - To multiply one exponent by another, we add exponents. To divide one power by another, of the same letter, we subtract the exponent of the divisor from the exponent of the dividend.
3. Divide
a2m5z3
by
m2z3.
OPERATION:
DIVIDE WITH SIGN: a2 m5 z3 ÷ m2z3 = a2m3 DIVIDE WITH FRACTION BAR a2 m5 z3 ________ = a2m3 1 m2 z3
ANALYSIS -
Divide a2 by an implied one.
Any number divided by one equals itself.
In this case a2 ÷ 1 equals a2.
Therefore the quotient includes a2.
Divide m5 by m2.
The exponents of m equal 5 and 2.
2 subtracted from 5 equals 3.
Therefore m5-2 equals m3.
The quotient includes m3.
Divide z3 by z3.
Any number divided by itself equals one.
Or consider z 3 - 3 equals z0.
Any number to the power of zero also equals one.
The quotient so far equals a2m31.
Any number times one equals itself. Therefore drop the 1,
for the final quotient a2m3.
Page 53
Page 53
Help with Algebra Homework
Free tutorials provide math help for math problems. Tutorials start with algebra 1. Algebra tutorials include word problems related to algebra topics, as they're introduced. See the menu, above for algebra math equations, and explanation, by section.
Windows algebra games, designed to help with classwork, coming soon!
Windows games, in progress, work with this set of free tutorials, modified
from the public domain text book titled,
New Elementary Algebra
containing the rudiments of the science for schools and academies.
By Horation N Robinison, LL. D. Ivison, Blakeman & Company, Publishers, New York and Chicago.



