Trigonometry Textbook
Trigonometric Functions
Chapter 1.1
Read the introduction, then learn three important ratios representing sine, cosine and tangent.
Introduction
The study of trigonometry is simple and interesting. Do not allow either the sound or the size of the word to frighten you. From geometry you're familiar with the word polygon. A trigon is simply a three sided polygon, a triangle, and metry means measure. Hence the name Trigonometry.
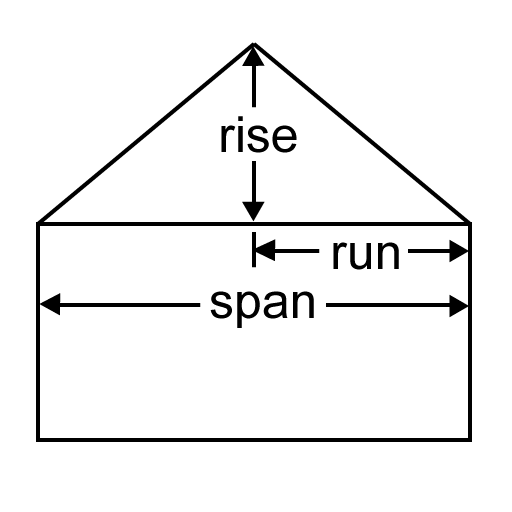
Recently a builder engaged in the construction of a house with a gabled roof, came to one of the authors of this book to ask a question in mathematics. The following conversation took place:
Builder: I want to know how to find the angle at which to pitch a gabled roof.
Author: That's simple. Do you know the rise? (See figure.)
Builder: Yes. Eight feet.
Author: What is the run or the span? (See figure.)
Builder: The span is 24 feet, so that the run must be 12 feet.
Author:
Convert the fraction 8/12 into the decimal 0.67.
Apply the
inverse function of the tangent; arctan(0.67).
The value returned, rounded up, equals 340.
The angle to pitch the roof is approximately 340.
The author was able to answer the question asked by the builder because he knew the dependence of one part of a triangle upon other parts. Other instances in which this dependence is used are found in the calculation of distances across swamps, lakes, forests and inaccessible places in general. This interdependence of the parts of a triangle may be used to find the heights of tall buildings or trees, the distances of ships at sea or of objects on a landscape, the altitude of the sun and of other stars. We may summarize by stating that for measurements which must be found indirectly, trigonometry is basic.
Besides the practical uses of trigonometry mentioned in the previous paragraph, and it's obvious use in surveying and navigation, trigonometry has indispensable use in mathematical analysis. Parts of analytical geometry and calculus would be impossible to understand without knowledge of plane trigonometry. Certain work in the solution of equations is greatly facilitated by the use of trigonometry in higher mathematics. To some people this use of trigonometry in mathematical analysis is of prime importance.
In plane trigonometry you will study the interesting relations of the sides and of the angles of triangles and of figures that can be decomposed into triangles. When you learn and understand these relations, you will better appreciate what is meant when we say that the sides and the angles of a triangle are interrelated.
Three Important Ratios
Let us begin the study of these relations by examining the right triangle
ABC in which the angle C is the right angle.
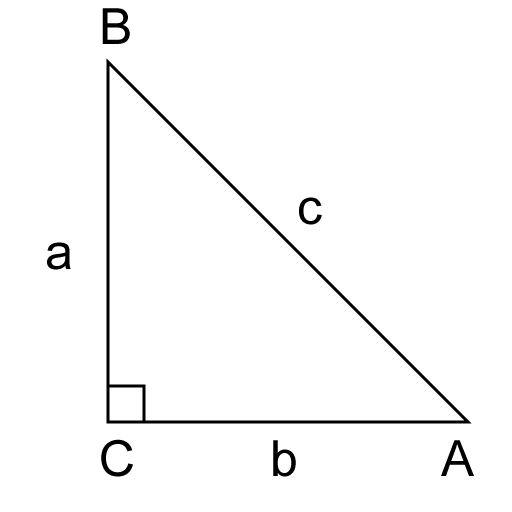
In the right triangle ABC, we call the hypotenuse, the side
opposite the right angle, c, and the two legs, a and b.
To distinguish between a and b, we speak of the opposite leg and
the adjacent leg, depending upon the angle to which we refer.
If we refer to angle A, then b is the adjacent leg and a,
the opposite leg. If we refer to angle B then a is the adjacent leg and b,
the opposite leg.
The ratio of the opposite leg to the hypotenuse is known as the sine (abbreviated sin) of an angle.
The sine of angle B is b divided by c. This is written as follows:
b
sin B = -
c
What ratio represents the sine of angle A?
The sine of the right angle C will be explained more fully later.
The ratio of the adjacent leg to the hypotenuse is known as the cosine of an angle. this is written as follows:
a
cos B = -
c
What ratio represents the cosine of angle A?
The ratio of the opposite leg to the adjacent leg is known as the tangent of an angle. For example:
b
tan B = -
a
What ratio represents the tan of angle A?
Pages 1-4
Trigonometry Help
Buttons link to trigonometry textbook sections.
Trigonometry helps programmers, engineers, scientists, teachers, and students. Trigonometry provides a foundation with mathematics required for game programming, science, technology, engineering, and math (STEM).
Trigonometry tutorials are modified from the public domain book titled,
Plane and Spherical Trigonometry
,
by Hayes and Leventhal.
Globe Book Company, New York, N. Y.
Love learning math with tutorials and games from Seven Thunders.



